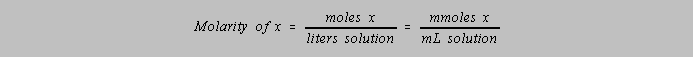
Chapter 2. Basic Concepts

A system of units and prefixes established to simplify and to unify communication between scientists.

Seven basic quantities	Unit	Abbreviation
Mass	kilogram	kg
Length	meter	m
Time	second	s
Temperature	kelvin	K
Amount of substance	mole	mol
Electric current	ampère	A
Luminous intensity	candela	cd

Mass. The kilogram (kg). The kilogram of a particular cylinder of platinum-iridium alloy, called the International Prototype Kilogram, which is preserved in a vault at Sèvres, France, by the International Bureau of Weights and Measures.

Length. The meter (m). The meter is the distance that light travels in a vacuum in 1/299 792 458 of a second.

Time. The second (s). The second is the unit of time of the International System of Units. The definition adopted at the October 13, 1967 meeting of the 13th General Conference on Weights and Measures is: "The second is the duration of 9,192,631,770 periods of the radiation corresponding to the transition between the two hyperfine levels of the fundamental state of the atom of cesium 133." The frequency (9,192,631,770 Hz) which the definition assigns to the cesium radiation was carefully chosen to make it impossible, by any existing experimental evidence, to distinguish the new second from the "ephemeris second" based on the earth's motion. Therefore no changes need to be made in data stated in terms of the old standard in order to convert them to the new one. The atomic definition has two important advantages over the previous definition: (1) it can be realized (i.e., generated by a suitable clock) with sufficient precision, ± 1 part per hundred billion (10¹¹) or better, to meet the most exacting demands of modern metrology; and (2) it is available to anyone who has access to or who can build an atomic clock controlled by the specified cesium radiation. (A description of such clocks is given in "Atomic Frequency Standards," NBS Tech. News Bull. 45, 8-11 (Jan., 1961). For more recent developments and technical details, see R. E. Beehler, R. C. Mockler, and J. M. Richardson, "Cesium Beam Atomic Time and Frequency Standards," Metrologia 1, 114-131 (July, 1965)). In addition one can compare other high-precision clocks directly with such a standard in a relatively short time -- an hour or so compared against years with the astronomical standard. Laboratory-type atomic clocks are complex and expensive, so that most clocks and frequency generators will continue to be calibrated against a standard such as the National Institutes of Standards and Technology (NIST) Frequency Standard, controlled by a cesium atomic beam, at the Radio Standards Laboratory in Boulder, Colorado. In most cases the comparison will be by way of the standard-frequency and time-interval signals broadcast by NBS radio stations WWV, WWVH, WWVB, and WWVL. There has been a recent proliferation of desk and wall clocks which tune into these stations a couple of times a day and reset themselves. Often they operate on an AA battery. When they first arrived on the mass market they cost around $400, but as in all things "hi-tech" the prices have fallen. Desk models can now be purchased for under $30. Come in to your instructor's office and see the wall-mount version.

Temperature. The Kelvin (K). The Kelvin, the unit of thermodynamic temperature, is the fraction 1/273.16 of the thermodynamic temperature of the triple point of water. The decision was made at the 13th General Conference on Weights and Measures on October 13, 1967 that the name of the unit of thermodynamic temperature would be changed from degree Kelvin (symbol: ^oK) to kelvin (symbol: K). The name (kelvin) and symbol (K) are to be used for expressing temperature intervals. The former convention which expressed a temperature interval in degrees Kelvin or, abbreviated, deg. K is dropped. However, the old designations are acceptable temporarily as alternatives to the new ones. One may also express temperature intervals in degrees Celsius.

Amount of substance. The mole (mol). The mole is the number of atoms of C¹² in 12.0000 g of C¹².

Electrical current. The ampère (A). The ampère (unit of electric current) is the constant current which, if maintained in two straight parallel conductors of infinite length, of negligible circular sections, and placed 1 meter apart in a vacuum, will produce between these conductors a force equal to 2 x 10^-7 newton per meter of length.

Luminous intensity. The candela (cd). The candela is the luminous intensity, in the direction of the normal, of a black body surface 1/600,000 square meter in area, at the temperature of solidification of platinum under a pressure of 101,325 newtons per square meter.

Exponent Prefixes

Factor	Prefix	Symbol
10²⁴	yotta	Y
10²¹	zetta	Z
10¹⁸	exa	E
10¹⁵	peta	P
10¹²	tera	T
10⁹	giga	G
10⁶	mega	M
10³	kilo	k
10²	hecto	h
10¹	deka	da
10^-1	deci	d
10^-2	centi	c
10^-3	milli	m
10^-6	micro	µ
10^-9	nano	n
10^-12	pico	p
10^-15	femto	f
10^-18	atto	a
10^-21	zepto	z
10^-24	yocto	y

These are the seven fundamental base units and accompanying prefixes. All of the other units may be derived from these -- they are called derived units.

A mole of molecules is Avogadro's number of molecules. Avogadro's number is the number of

¹²C atoms in 12.0000 g of ¹²C and has been experimentally determined to equal 6.022 x 10 ²³.

Add up the atomic weights multiplied by the number of atoms of each element in the compound to get 60.05 g/mol.

Exercise 2-2a. How many moles of citric acid, C₆H₈O₇, MW=192.14, are contained in 6.00 g of the pure acid?

Exercise 2-2b. How many millimoles of citric acid are contained in 6.00 g of the pure acid?

Exercise 2-3a. How many grams Na⁺ are contained in 32.7 g trisodium phosphate, Na₃PO₄ 10 H₂O, F.W. 344.09?

Exercise 2-3b. How many grams of the phosphate ion, PO₄^3-, are contained in the amount given above?

The following concentrations and concentration relationships are of importance to and will often be found in studies involving the quantitative analyses of chemical substances:

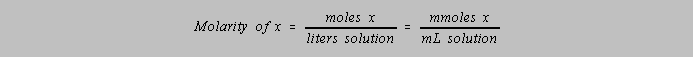
Exercise 2-4. Given a 1.25 liter solution which contains 3.74 g urea, H₂NCONH₂, MW=60.06, determine the molar concentration of urea in this solution.

Regarding solution concentration, one speaks of analytical molarity, or that which would be present if the substance does not enter into reactions which change the concentration of the species under discussion, and equilibrium molarity of a species which reflects the disappearance of part of the original species due to dissociation, complex formation or other equilibria processes.

Exercise 2-5a. Determine the analytical molarity of 11.27 g anhydrous sodium sulfate, Na₂SO₄, in 500.00 mL solution.

Exercise 2-5b. Determine the equilibrium molarity of Na⁺ and SO₄^2-, written [Na⁺] and [SO₄^2-], in the solution described above.

Exercise 2-5c. A solution of acetic acid, CH₃COOH, MW=60.05, having an analytical molarity of 0.100 M has what equilibrium concentration of hydronium ion, H₃O⁺, if the dissociation goes according to the equation and the molecular form of the acid is 5% ionized?

Exercise 2-5d. Determine the species molarity of CH₃COO^- for the solution described above.

The student of Quantitative Analysis is often asked to prepare a solution of some given analytical molarity.

Exercise 2-6a. Explain how one would prepare 50 mL of a 0.250 M solution of sodium sulfate decahydrate,

(To be performed in class. First the quantity is determined, then one is asked what exactly is the procedure which one follows.)

One might be asked the question above in a form which requires the weight of reagent necessary to produce a given species molarity:

Exercise 2-6b. Explain how one would prepare 50 mL of a 0.125 M solution of sodium ion, starting with the reagent sodium sulfate decahydrate, Na₂SO₄ 10 H₂O, MW=322.19, assuming 100% dissociation of sodium ion.

Percent concentration. Percent concentration may be thought of as parts per hundred.

Weight percent is often used to express the concentration of commercial reagent grade acids. Concentrated aqueous ammonia is sold as 28% (w/w) NH₃. (See the Web link

10% aqueous butanol, for example, would be a solution in which 10 mL pure butanol is diluted to give 100 mL solution.

Wt/vol solutions are often dilute aqueous solutions in which the calculation involved takes advantage of the fact that the density of water is close to 1 g/mL. Dilute w/v solutions have concentrations very close to the values which would be reported for their w/w concentrations. At higher concentrations there is a greater divergence. For example, 50% w/w NaOH is 76.3% (w/v) NaOH because the density of the solution rises significantly above 1.00 g/mL

The use of (w/w)% for (weight/weight)% is an historical artifact which is still seen from time to time. A better designation would be (m/m)% for (mass/mass)% but because the abbreviation "m" is that which is used for the metric unit of length, (w/w) has stuck around far longer than is justifiable. To get around the use of (w/w), companies which produce reagent grade acids simply say "Assay". That is, on a bottle of Spectrum® reagent grade sulfuric acid one finds the designation "Assay (H₂SO₄ ). . . . . . . 95.0 - 98.0 %". That designation very definitely describes a percent concentration found by the operation

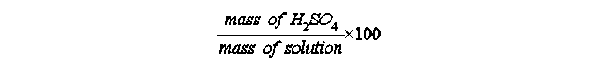
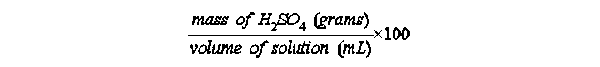
Exercise 2-7. Knowing the above values for the (w/w) and (w/v) solution of NaOH, determine the density of that solution.

Consider the following table of solution densities at 20^oC:

Solution	Density
10% (w/w) KCl	1.08 g/cc
10% (w/w) NaCl	1.07 g/cc
10% (w/w) KOH	1.08 g/cc
10% (w/w) KCl	1.06 g/cc
10% (w/w) BaCl₂ 2H₂O	1.082 g/cc

Exercise 2-8. Determine the (w/v) value for the 10% (w/w) solution of KOH above.

Parts per million (ppm). Parts per million is a concentration convention convenient to use for very dilute solutions.

Exercise 2-9. Determine the ppm of ferrous ion, Fe²⁺, in a solution known to be 1.2 × 10^-6 M Fe₃(PO₄)₂ 8H₂O

When diluting solutions, a directive may specify a 1:5 volume/volume dilution. Although this usually means 1 volume of concentrated solution to 5 volumes of the final dilute solution, that might not be the case depending upon the procedure in any particular laboratory. The student is advised always to verify whether the meaning might be 1 volume of concentrated solution to 5 volumes of water. Making a mistake in the preparation of the final solution can lead to serious consequences particularly where the preparation of nutrient solutions in experimentation on humans and animals is involved.

Exercise 2-10. Determine the volume/volume ratio needed to dilute a 0.5 M saline solution to 0.2 M.

p-functions The p-function is the negative logarithm to the base 10 of the molarity of a given species.

Exercise 2-11a. Given a solution known to be 3.7 × 10^-4 M NaCl and 4.5 × 10^-3 M HCl , determine pH, pNa and pCl for these solutions.

Exercise 2-11b. Given a solution with a pOH of 4.37, determine the species molarity of the OH^- ion.

Today with modern hand calculators, converting X to log₁₀X is a matter of a single keystroke. Converting a p function to a concentration is equally simple because of the following relationship.

Exercise 2-12. Consider pAg in a solution of silver nitrate, AgNO₃, to equal 6.74. What is the molarity of Ag⁺ ?

Since the statement y=log₁₀X could be identically rewritten as X=10^y, the expression above could also be rewritten as

The numeric value for a density given as g/mL or kg/L is the same. Water with a density at 20^oC of 0.998 g/mL has a density of 0.998 kg/L. The density of gases is usually given as g/L because at normal pressures the density is about a thousand times less than that of liquids. The density of dry air at 760 torr at 1 atm pressure is 1.185 g/L.

The specific gravity is a ratio between the mass of a given volume and the mass of an equal volume of water at 4^oC. Since the density of water at 4^oC is practically 1.00 g/cc, the density and specific gravity of aqueous solutions are almost identical. The difference needs always to be considered for analytical work of high precision, however.

Exercise 2-13. Commercial reagent grade formic acid is 90% w/w and has a specific gravity of 1.20. Determine the molarity of formic acid in a bottle of commercial reagent grade formic acid.

Given any two of the following three: (w/w)%, molarity and specific gravity, one can calculate the third. Given just the molarity, one is able to calculate methods of diluting solutions volumetrically.

Exercise 2-14. Hydriodic acid, HI, is sold in reagent grade bottles as 57% (w/w) HI and has a specific gravity of 1.70. Explain how you would prepare 100 mL of 1.25 M hydriodic acid, using only a 100 mL volumetric flask and a 20 mL graduated pipette.

Going from step 2. to step 3. requires a stoichiometric ratio which is usually determined by balancing a chemical equation.

Exercise 2-15a. Calcium oxalate, CaC₂O₄ , can be precipitated by adding to a solution of calcium hydroxide, Ca(OH)₂, sufficient sodium oxalate, Na₂C₂O₄ , to react with all calcium hydroxide present. What mass sodium oxalate would be required to precipitate all of the calcium in a solution containing 3.74 g Ca(OH)₂?

25.0 mL 0.100 M Ca(OH)₂ is added to 20.0 mL 15.8 % (w/v) Na₂C₂O₄. Calculate the weight CaC₂O₄ formed.

Exercise 2-16b. What is the equilibrium concentration in molarity of all ionic species remaining after the reaction?

Disclaimer: The views and opinions expressed on unofficial pages of California State University, Dominguez Hills faculty, staff or students are strictly those of the page authors. The content of these pages has not been reviewed or approved by California State University, Dominguez Hills.