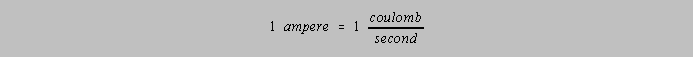
Chapter 4, Titrimetry
4-1. Definitions. Titrimetry refers to that
group of analytical techniques which takes advantage of titers or concentrations of solutions. In
metallurgy the word "titer" refers to the fineness of gold or silver. In chemistry it is the measure
of the concentration of a solution. In medicine it is defined as the extent to which an antibody
solution can be diluted before it ceases to give a positive reaction with an antigen.
Though in chemistry the term titrimetry often refers to the use of some volume of a solution
of known concentration
to determine the quantity of analyte, there are still some variations on the use of the term. It is
used rather to denote
a quantity of some other measurement parameter which relates directly to the quantity of analyte
which is to be
measured:
Volumetric titrimetry establishes a quantity of analyte using
volumes of reagents of known concentrations and the
knowledge of the stoichiometry of the reactions between the reagents and the analyte(s).
Gravimetric titrimetry determines the quantity of analyte by a
measure of the mass of a solution of known
concentration.
Coulometric titrimetry arrives at the amount of analyte by
measuring the duration of a given electrical current. Since
amperes x time = coulombs or total charge, the number of equivalents of analyte can be
measured
by relating the
extent of reaction to the number of moles of electrons (Faradays).
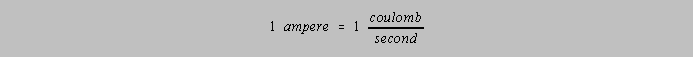
![]()
![]()
The equivalence point is the point at which a volume, a mass
or a quantity of charge equivalent to the amount of
analyte present in the sample to be measured is reached. It is the point of stoichiometric
chemical
equivalence.
The end point is the point at which some detection technique tells you that chemical equivalence has been reached. The end point may occur before or after the equivalence point, giving a titration error. It is for this reason that blank samples are often used. Blank samples are prepared so that you have a measure of the amount that needs always to be added to or subtracted from the end point (the titration error) to achieve the equivalence point.
4-2. Primary and Secondary Standard Solutions
In volumetric titrimetry one uses a standard solution the
concentration of which is known with great precision and
which reacts stoichiometrically with the analyte. Standard solutions are referred to either as
primary standards or
secondary standards. Primary standards can be prepared by
weighing directly and dissolving to a measured volume
the reagent which is to react with the analyte. But primary standards must meet stringent
requirements:
1. High purity
2. Stability in presence of air
3. Absence of any water of hydration which might vary with changing humidity and temperature.
4. Cheap
5. Dissolves readily to produce stable solutions in solvent of choice
6. A larger rather than smaller molar mass
These conditions are met by few materials. Anhydrous sodium carbonate, silver nitrate,
potassium hydrogen
phthalate are a few which do meet these conditions. The National Institute of Standards and
Technology (NIST),
formerly the National Bureau of Standards publishes lists of and is a reliable source of
exhaustively analyzed primary
standards. Moreover, its NIST Chemistry Web book is a useful source of data on many inorganic
and organic
compounds.(1)
4-3. Criteria for Practical Use of Standard Solutions
So as to be useful as a measure of the quantity of analyte in a sample, a standard solution
must meet four criteria:
1. The reagent in the solution must have sufficient stability so that its concentration need be determined only once. Any deterioration in strength due to reaction with components of water or air would make the reagent unacceptable.
2. The reagent in the solution must react rapidly with the analyte. Slow approaches to equilibrium can cause erroneous judgments about reaction completeness.
3. The reaction of the analysis must occur with a completeness easily detectable by an appropriate indicator.
4. The reagent must react with the analyte in a simple and stoichiometrically predictable
manner. Any side reactions
would render a reagent unacceptable.
Two excellent standard solutions are those of hydrochloric acid, HCl and potassium
hydrogen
phthalate, KHC8H4O4.
Three others which have some instability but with appropriate precautions have proven to be
excellent standard
solutions are sodium thiosulfate, Na2S2O3 (light
sensitivity, susceptible to bacterial oxidation), silver nitrate, AgNO3
(light sensitivity) and potassium permanganate, KMnO4 (water oxidation catalyzed
by light, heat, Mn2+ and MnO2).
4-4. Determination of the concentration of a standard solution.
The direct method of solution standardization is the obvious
choice if the reagent is a primary standard which meets
all of the criteria described above and whose weight offers a repeatable observation proportional
to the number of
moles of substance expected. Solutions of sodium carbonate and silver nitrate can be prepared in
this manner.
The method of standardization can be used if a primary
standard reacts quantitatively with the reagent needed in
the standard solution. HCl cannot be considered to be a primary standard because of its gaseous
form at room
temperature, but its solutions may be standardized against anhydrous
Na2CO3.
For the purposes of this course, concentration in molarity will
be given the symbol of c and that of normality
the
symbol cN. The following two relationships will be useful:
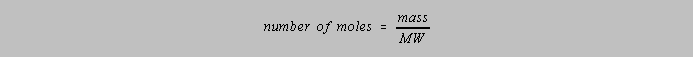
and
![]()
![]()
4-5. Typical problems in volumetric titrimetry:
Example 4-1. Explain how to prepare 2.000 L of 0.1374 M potassium sulfate, K2SO4.
(To be executed in class. Execution will include discussion of odd molarity, large volume
and
actual technique
necessary to get the job done.)
Example 4-2a. Highly purified sodium tetraborate decahydrate (Borax, would you believe
it?), Na2B4O710H2O may
be used as a primary standard. The neutralization equation is
![]()
The equivalence point for this reaction occurs at pH 7-8. Explain how to prepare 250.00 mL of 0.0100 N Na2B4O710H2O solution. What is the molarity of Na+ ions?
(To be executed in class. Execution will include discussion of equivalent weight, the
conversion of normality and
molarity and the actual technique one might use to prepare the solution.)
Example 4-2b. Describe how you could prepare 100.00 mL portions, starting from the solution above, of 0.00500 M, 0.00200 M and 0.00100 M Na+.
(To be executed in class. Execution will include discussion of techniques of dilution and the
use of the equation
![]()
Example 4-3. Describe how to prepare 500 mL of a solution of formic acid having a concentration of 0.25 M starting with commercial grade formic acid. Refer to the table in the appendix for properties of the commercial grade acid.
(To be executed in class. Execution will include discussion of assumed precision conveyed
by
given data and the
technique of preparation, maintaining awareness that concentrated acids ought
never to be
weighed on an analytical balance.)
4-6. Problems which require the amount (moles) and a stoichiometric
ratio.
Given the three concepts of
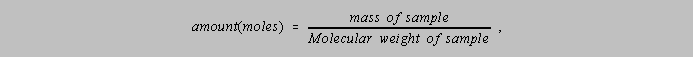
![]()
and the stoichiometric ratio given by some relevant equation, be able to solve problems like
the following:
Example 4-4. 0.3527 g anhydrous sodium carbonate requires 38.47 mL HCl solution to achieve a bromocresol green end point after a procedure identical to that which you will perform in laboratory. The blank correction is found to be -0.05 mL. Determine the molarity of the acid solution.
(To be executed in class. Execution will include a discussion of the stoichiometry of the
reaction, the necessity of
boiling your solution just before reaching the final end point and the blank correction.)
Example 4-5. Sodium oxalate, Na2C2O4, is used as
a
primary standard for the standardization of potassium
permanganate solution according to the equation
![]()
If 0.1847 g Na2C2O4 requires 44.57 mL KMnO4 solution to an end point produced by the intense violet red color, calculate the molarity of the potassium permanganate solution.
(To be executed in class. Execution will include a discussion of the progression
grams ---> moles --> stoichiometric ratio ---> moles ---> molarity.)
Example 4-6. A standard assay for iron in iron ore can be accomplished by dissolving the
ore
in concentrated acid,
reducing the resulting Fe3+ to Fe2+ and titrating with permanganate to
a red-violet end point according to the equation
![]()
If a 0.7248 g sample of ore requires 38.92 mL of 0.02897 M KMnO4 solution to arrive at the end point, calculate (a) the %Fe in the sample and (b) the %Fe3O4 in the sample.
(To be executed in class. The execution will include a discussion of the features of Example
4-5 above plus the added
point about reporting an analyte in a sample in a purely hypothetical form.
Example 4-7. The determination of total nitrogen in proteinaceous matter, either plant or animal, can be carried out by means of the Kjeldahl method which involves digestion of the sample in concentrated sulfuric acid, often with a mercury catalyst (which is why the Kjeldahl method is used less and less nowadays), neutralization of the acid while cooled on ice by slow (!) addition of small pellets of solid NaOH. The process requires some skill so as not to crack the flask owing to mini-explosions caused at least initially by the small shock waves produced during the NaOH addition. In any case, at last when the solution is made basic, heating it causes the resulting ammonia to be forced out, according to the equation
![]()
The ammonia is distilled from the original flask into a second which contains a known
volume
of standardized H2SO4,
partially neutralizing the H2SO4, according to the equation
![]()
The remaining H2SO4 must be brought to an end point using
standardized NaOH solution and a bromocresol green
end point.
Calculate the %N in a .4076 g sample of oleander root extract if the ammonia after digestion is distilled into 100 mL 0.0236 M H2SO4 and final titration with 0.0527 M NaOH requires 22.38 mL of the base to achieve a phenolphthalein end point.
(To be executed in class. The execution will include a discussion of the total moles of
H2SO4 reacting with the
summation of moles of NH3 and NaOH.)
4-7. Sygmoidal Titration Curves
For the purpose of demonstrating the origin of sygmoidal titration curves, even the necessity
of portraying titration
curves as sygmoidal, it is instructive to look at what happens to the hydronium ion concentration
during the titration
of a strong acid with a strong base. Consider the titration of 50.00 mL 0.1000 M NaOH with
0.1000 M HCl. The
hydronium ion concentration remains quite low almost to the equivalence point. As all of the
hydroxide ion is used
up, the hydronium ion concentration suddenly increases by 4-5 orders of magnitude within a
volume change of a few
hundredths of a mL. This translates to 4-5 pH units and illustrates why titration curves must be
portrayed in a log-linear manner. Consider the following table (the student is invited to fill in the
blank cells:
| mL 0.1000 M
HCl |
mmoles HCl
added |
Total volume
(mL) |
mmoles OH-
remaining |
mmoles H3O+
in excess |
[OH-] | [H3O+] | pH |
| 0.00 | 0 | 50.00 | 5.000 | 0.100 | 1x10-13 | 13 | |
| 40.91 | 4.091 | 90.91 | 1x10-2 | ||||
| 49.01 | 99.01 | 0.099 | 1x10-3 | ||||
| 49.90 | 4.990 | .01 | 1x10-4 | ||||
| 49.99 | 4.999 | 99.99 | .001 | 1x10-5 | |||
| 50.01 | 5.001 | 0.001 | 1x10-9 | 10-5 | |||
| 50.10 | 5.010 | 100.10 | 1x10-10 | 10-4 | |||
| 50.99 | 5.099 | 100.99 | |||||
| 59.09 | 5.909 | 109.09 | 0.909 | 1.25 x 10-12 | 8.3x10-3 | ||
| 100.00 | 10.00 | 150.00 | 5.00 | 3x10-13 | 0.033 |
Now plot pH vs volume of HCl added:
+ + + + + + + + + + + + + + + + + + + + + + + + + + + + + + + +
+ + + + + + + + + + + + + + + + + + + + + + + + + + + + + + + +
+ + + + + + + + + + + + + + + + + + + + + + + + + + + + + + + +
+ + + + + + + + + + + + + + + + + + + + + + + + + + + + + + + +
+ + + + + + + + + + + + + + + + + + + + + + + + + + + + + + + +
+ + + + + + + + + + + + + + + + + + + + + + + + + + + + + + + +
+ + + + + + + + + + + + + + + + + + + + + + + + + + + + + + + +
pH+ + + + + + + + + + + + + + + + + + + + + + + + + + + + + + + +
+ + + + + + + + + + + + + + + + + + + + + + + + + + + + + + + +
+ + + + + + + + + + + + + + + + + + + + + + + + + + + + + + + +
+ + + + + + + + + + + + + + + + + + + + + + + + + + + + + + + +
+ + + + + + + + + + + + + + + + + + + + + + + + + + + + + + + +
+ + + + + + + + + + + + + + + + + + + + + + + + + + + + + + + +
+ + + + + + + + + + + + + + + + + + + + + + + + + + + + + + + +
+ + + + + + + + + + + + + + + + + + + + + + + + + + + + + + + +
+ + + + + + + + + + + + + + + + + + + + + + + + + + + + + + + +
Volume HCl added (mL)
This is the shape of a sygmoidal titration curve.
At and near the equivalence point, two phenomena affect the pH: (1) the stoichiometry of the
chemical equation and
(2) the autoionization or autoprotolysis of water. As the amount
of HCl added approaches the equivalence point,
the total amount of OH- decreases by virtue of the stoichiometry of the reaction, but
as that amount approaches zero
molarity, the amount always present due to autoprotolysis becomes more and more important.
As
the equivalence
point is approached in an acid-base titration the concentration of the species in excess continues
to decrease.
Throughout the titration, on either side of the equivalence point and quite close to it the pH can
be estimated with
very good precision by simple arithmetic. For example, in the titration of 50 mL of 0.1000 M
NaOH with 0.1000
M HCl discussed above, the cells of the table showing various values of amount, volume and
concentration can be
calculated using only addition, subtraction, multiplication and division. But when the excess
OH- becomes very small,
its value approaches that which is produced by protoloysis, that is,
![]()
This process governs
the value of the protolysis equation:
![]()
The simple method of
estimating the value of the hydronium ion and hydroxide ion concentrations then is
complicated by this additional source of acidic and basic species, and the calculation moves into
the realm of the
quadratic equation.
The general form for a quadratic equation can be written as
![]()
The
quadratic formula shows the root, or value of x for the quadratic equation:
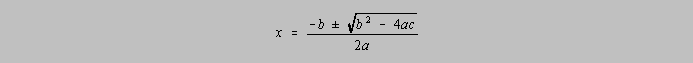
Note in the table
above that as HCl is added, the mmoles of OH- decrease toward zero, but until the
equivalence
point is approached that value greatly exceeds the mmoles of OH- which are
produced by protolysis. For example,
in the table above for the addition of 49.99 mL HCl ,
| mL 0.1000 M
HCl |
mmoles HCl
added |
Total volume
(mL) |
mmoles OH-
remaining |
mmoles H3O+
in excess |
[OH-] | [H3O+] | pH |
| 49.99 | 4.999 | 99.99 | 0.001 | //////// | 1 x 10-5 | 1 x 10-9 | 9 |
all calculations are accomplished by addition, subtraction and division. But look what
happens if that process is
carried to the following logical absurdity:
| mL 0.1000 M
HCl |
mmoles HCl
added |
Total volume
(mL) |
mmoles OH-
remaining |
mmoles H3O+
in excess |
[OH-] | [H3O+] | pH |
| 49.99 | 4.999 | 99.99 | 0.001 | //////// | 1x10-5 | 1x10-9 | 9 |
| 49.999 | 4.9999 | 99.999 | 0.0001 | //////// | 1x10-6 | 1x10-8 | 8 |
| 49.9999 | 4.99999 | 99.9999 | 0.00001 | //////// | 1x10-7 | 1x10-7 | 7 |
| 49.99999 | 4.999999 | 99.99999 | 0.000001 | //////// | 1x10-8 | 1x10-6 | 6 |
But the result is ridiculous. On the one hand the equivalence point has not yet been reached. The solution ought still to be a little basic but the pH is calculated to be 6. This paradox arises because the protolysis leading to hydroxide and hydronium ions has been ignored. That is, the calculation of excess hydroxide ion by the simple arithmetic process is in error. If we let x = the mmoles of H3O+ produced by protolysis we have to admit that x moles of OH- are also produced by protolysis, because stoichiometrically the ratio of production is 1:1, according to the protolysis equation
![]()
Then the
total number of mmoles of OH- present, for the last
row above is 0.000001 + x
Since the total volume is nearly 100.0 mL, the protolysis equation becomes
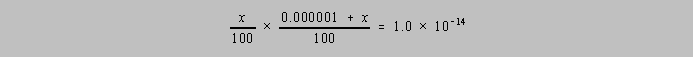
which simplifies to
the following quadratic form:
![]()
The value of x (millimoles of H3O+) is found by solving the
quadratic formula,
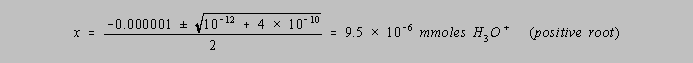
to yield
[H3O+] = 9.5 x 10-8 , giving a pH of 7.02, just slightly
basic, as one would expect if the titration with HCl
hasn't quite reached the equivalence point.
Exercise. Carry out this calculation for mmoles OH- = 0.0001 and 0.00001.
All this is by way of saying that when one approaches the equivalence point, the pH cannot
be
estimated without the
use of a quadratic equation.
4-8. The Henderson-Hasselbalch Equation
A weak acid, symbolized by the formula HA, hydrolyzes (reacts with water) according to an equation showing equilibrium between its acidic and basic forms:
We say that the acidic form is HA and the basic form A-. The law of mass action after Guldberg and Waage predicts that the equilibrium constant for this process can be expressed as
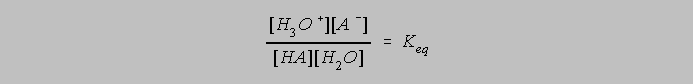
The designation of
molar concentrations is an approximation to the species activity, a value which diverges from the
molarity as the concentration increases. Since our concentrations in such calculations rarely
approach even 1 molar,
we shall follow this approximation. Equally important, the concentration of pure water is 55.6 M
and varies little
when solutes at concentrations in the vicinity of 0.01 to 1.0 M are introduced. That is, the
concentration (and
activity) of water is nearly constant where dilute solutions are involved, so the equation above
can
be rewritten as
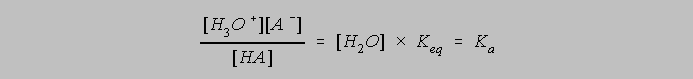
and Ka
is defined to be the acid dissociation constant.
If x=y, then log10x=log10y and we can effect the following modification of the equation above
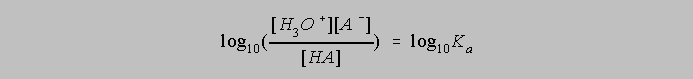
The left side can be
expanded to be
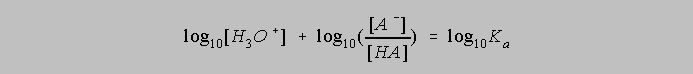
Multiplying both sides by -1 gives us
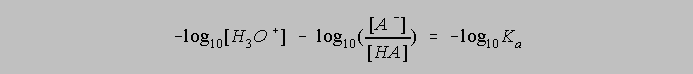
The p function of X, that is, pX is defined as -log10X, so the equation above can be rewritten as
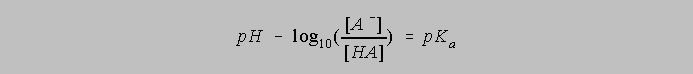
And this can be rearranged to give us the standard form of the Henderson Hasselbalch Equation:
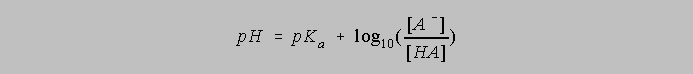
The Henderson Hasselbalch Equation often evokes strong negative responses from many teachers of chemistry. "The Henderson Hasselbalch Equation is a terrible crutch and I don't teach it," is a comment not infrequently heard. Yet the equation contains no approximations other than the fundamental one that molar concentrations approximately equal species activities. There is of course the matter of hydrolysis. In addition to the dissociation of a weak acid shown above, the conjugate base of that weak acid is often conveniently available as the sodium or potassium salt, NaA or KA, and the dissociation of NaA, for example, in an aqueous environment, follows the path
![]()
But A- (aq) hydrolyzes to some extent:
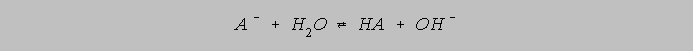
The hydrolysis of HA and of A- cause the original analytical molarities to change slightly to their species equilibrium molarities. The primary objection to the Henderson Hasselbalch Equation seems to focus on the approximation that the species equilibrium molarities equal the analytical molarities. In general, this is a valid objection. On the other hand, for weak acids with values of Ka on the order of 10-5 and less, the analytical molarities give a very close approximation to the values which can be used in the Henderson Hasselbalch Equation.
Example 4-8. Benzoic acid has an acid dissociation constant, Ka, equal to 6.31 x 10-5 . A buffer solution is known to have the following equilibrium concentrations:
[C6H5COO- ] = 0.0937 M
[C6H5COOH ] = 0.1443 M
Predict the pH of this buffer solution. (To be solved in class with attention paid to this exhibiting the simplest and most obvious of calculations applying the Henderson Hasselbalch Equation.)
Example 4-9. A CHE230 Student wishes to make up a buffer solution having a pH = 6.4 using the buffer pair of potassium acetate and acetic acid. Acetic acid has a Ka = 1.82 x 10-5 . Calculate the conjugate base/acid ratio which would be necessary to achieve this pH. (To be solved in class with attention being paid to this problem illustrating an answer one step away from determining the mass of each reagent necessary to achieve the desired pH.)
Example 4-10. Determine the mass of potassium acetate and volume of glacial acetic acid necessary to achieve the pH in Example 4-9 above if the total [acetate] + [acetic acid] concentration is to equal 0.1 M and the volume of the buffer solution is to equal 1.00 L.
1. United States Department of Commerce, NIST Chemistry Web Book,
http://webbook.nist.gov/chemistry/